如图，在三棱锥$P-ABC$中，$\triangle PBC$为等边三角形，点$O$为$BC$的中点，$AC\bot PB$，平面$PBC\bot $平面$ABC$.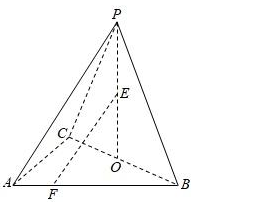


（1）求直线$PB$和平面$ABC$所成的角的大小.
（2）求证：平面$PAC{\bot }$平面$PBC$.
（3）已知$E$为$PO$的中点，$F$是$AB$上的点，$AF=\lambda AB$.若$EF// $平面$PAC$，求$\lambda $的值.

$(1)$${60}^{\\circ }$；

$(2)$证明见解析；

$(3)$$\\dfrac{1}{4}$

$(1)$$\because \triangle PBC$是等边三角形，点$O$为$BC$的中点，
$\therefore PO\bot BC$，
$\because 平面PBC\mathrm{\bot }平面ABC$，$平面PBC\cap \mathrm{平面}ABC=BC$，
$PO\subset 平面PAC$，$\therefore PO\bot \mathrm{平面}ABC$，
$\therefore \angle PBC$是直线$PB$和平面$ABC$所成角，
$\because \triangle PBC$是等边三角形，$\therefore \angle PBC={60}^{\circ }$，
$\therefore $直线$PB$和平面$ABC$所成角为${60}^{\circ }$.
$(2)$由上一题得$PO\mathrm{\bot }平面ABC$，
$\because AC\subset 平面ABC$，$\therefore PO\bot AC$，
$\because AC\bot PB$，$PB\subset \mathrm{平面}PBC$，$PO\subset 平面PBC$，$PB\cap PO=P$，
$\therefore AC\bot \mathrm{平面}PBC$，
$\because AC\subset 平面PAC$，$\therefore 平面PAC\mathrm{\bot }平面\mathrm{P}BC$.
$(3)$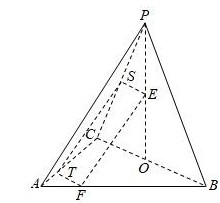

过点$E$，$F$分别作$ES// BC$，$FT// BC$，交$PC$，$AC$于$S$，$T$，连接$ST$，则$ES// FT$，
$\therefore $直线$ES$与直线$FT$确定一个平面，
$\because EF// \mathrm{平面}PAC$，$EF\subset 平面EST\,F$，
$平面PAC\cap 平面EST\,F=ST$，
$\because EF// ST$，
$\therefore $四边形$ESTF$是平行四边形，$\therefore ES// FT$，
$\because $$E$为$PO$的中点，$ES// BC$，$ES=\dfrac{1}{2}OC$，
$\because $$O$是$BC$的中点，$\therefore ES=\dfrac{1}{4}BC$，
$\because FT// BC$，$\therefore \dfrac{AF}{AB}=\dfrac{FT}{BC}=\dfrac{ES}{BC}=\dfrac{1}{4}$，
$\therefore \lambda =\dfrac{1}{4}$.